コンプリート! log(1 x)/x limit 239464-Log(1+x)/x limit
Integrate log(1tan x) from 0 to pi/4 Find the answer to this question along with unlimited Maths questions and prepare better for JEE examDerivatives Derivative Applications Limits Integrals Integral Applications Integal Approximation Series ODE Multivariable Calculus Laplace Transform Taylor/Maclaurin Series Fourier Series \log 1 2 3\pi e x^{\square} 0 \bold{=} Go Related » Graph » Number Line » Examples » Correct Answer ) {1}{1x}, 0 en RelatedOct 23, 14 · Using this fact, you can use the definition of the derivative as log (1x) log (1)/x as x tends to 0 Log (1) = 0, so you're left with the expression I was trying to work with log (x1)/x as x tends to 0 From that we can say that the limit is equal to the derivative of log at 1, which is 1
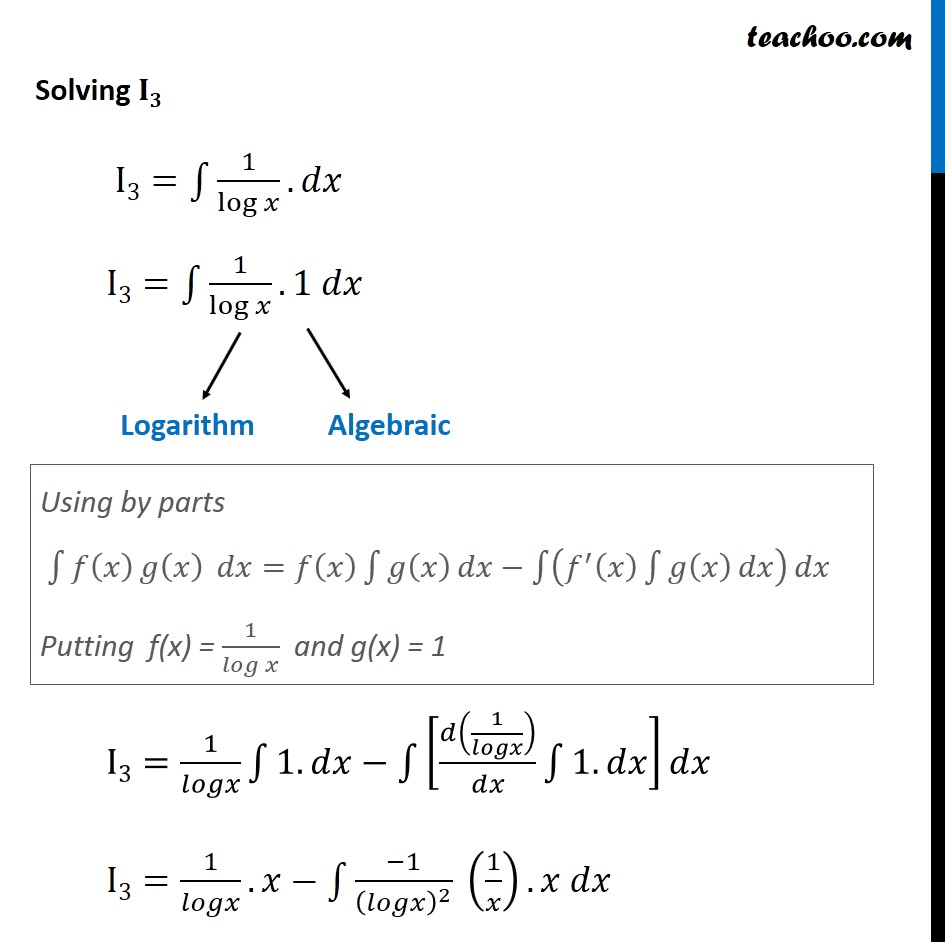
Example 40 Evaluate Log Log X 1 Log X 2 Dx Examples
Log(1+x)/x limit
Log(1+x)/x limit-Jan 02, 17 · Use that $\dfrac{1}{1x}=1xx^2x^3\ldots\quad (xLimit Calculator limit as x approaching 1 of (x^21)/(x1) Derivatives First Derivative;



Solved Exercice1 A Compute The Following Limits Log X2 Chegg Com
Logarithm base change rule log b ( x) = log c ( x) / log c ( b) Derivative of logarithm f ( x) = log b ( x) ⇒ f ' ( x) = 1 / ( x ln ( b) ) Integral of logarithm ∫ log b ( x) dx = x ∙ ( log b ( x) 1 / ln ( b) ) C Logarithm of negative number log b ( x) is undefined when x ≤ 0 Logarithm of 0Dec , 19 · Ex 711, 8 By using the properties of definite integrals, evaluate the integrals ∫_0^(𝜋/4) log(1tan𝑥 ) 𝑑𝑥 Let I=∫_0^(𝜋/4) log〖 (1tanLim ((x h)^5 x^5)/h as h > 0;
Limit (a^x−1)/x=log a as x approaches zero Mad Teacher Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't begin shortly, try restarting your device Up NextEvaluate limit as x approaches 0 of ( natural log of 12x)/x Evaluate the limit of the numerator and the limit of the denominator Tap for more steps Take the limit of the numerator and the limit of the denominator Evaluate the limit of the numerator Tap for more steps Take the limitIn THIS ANSWER and THIS ONE I showed, without the use of calculus, that the logarithm function satisfies the inequalities \frac{x1}{x}\le \log(x)\le x1 Therefore, we can write for x>1 \frac1x \le \frac{\log(x)}{x1}\le 1
The last limit is often summarized as "logarithms grow more slowly than any power or root of x" Derivatives of logarithmic functions edit d d x ln x = 1 x , {\displaystyle {d \over dx}\ln x={1 \over xI =log(1cosx ) in limit 0,π (1) use f(x ) =f(π0 x ) I =log(1cos(πx)) =log(1cosx ) (2) add equation (1) and (2)Integrate Log(1x)/ 1x 2 dx Upper Limit 1 Lower limit 0 Share with your friends Share 2



Visualization Of Various Limits



Evalute Limx 0 Log 1 Tan2x X X Belongs To Pi 8 Pi 8 Math Limits And Derivatives Meritnation Com
Limit (1 1/n)^n as n > infinity;As before, we use the exponential and natural log functions to rephrase the problem 1/x ln x 1 /x ln x x = e = e x Thus, lim x 1/x ln= lim e x x Since the function et is continuous, x→∞ x→∞ ln x ln x lim e x = e lim x→∞ x x→∞ ln x We can now focus our attention on the limitUsing the previous exercise (Section 69, Exercise #28)



Evaluate The Following Limit Lim X 0 Log 1 X 3 X 1
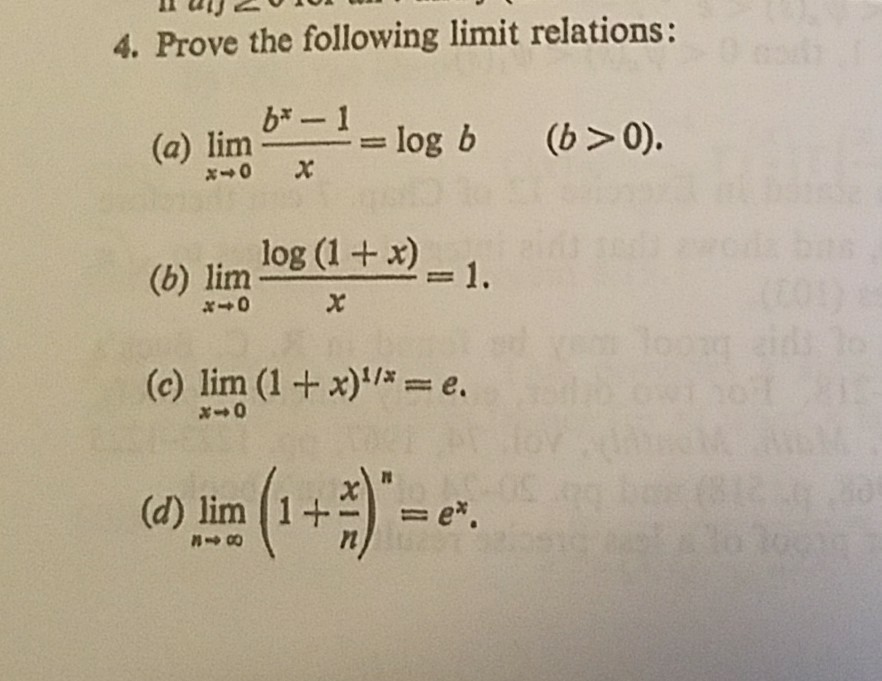


Solved 4 Prove The Following Limit Relations B 1 A Chegg Com
Expension of log(1x) is x (x^2)/2 (x^3)/3 ( x^4)/4 (Alternate signs) Applying the limit to all the inequalities in (3) as tends to , we have for the leftmost and rightmost terms Therefore, by the squeeze theorem* for the convergent sequencesDerivative of log(1x^2) by x = (2*x)/(x^21) Value at x= Derivative Calculator computes derivatives of a function with respect to given variable using analytical differentiation and displays a stepbystep solution It allows to draw graphs of the function and its derivatives Derivative calculator Integral calculator DefiniteLim x!0 lnx = 1 I We saw the last day that ln2 > 1=2 I Using the rules of logarithms, we see that ln2m = mln2 > m=2, for any integer m I Because lnx is an increasing function, we can make ln x as big as we



Lim X Rarr0 Log 1 X 2 Log Cos X



Lim Log 2 X Log 2 X Tanx Brainly In
Lim (x^2 2x 3)/(x^2 2x 3) as x > 3;Its very simplewe know that limit x tends to 0, log(1ax)/x=1REFER TO CLASS 11 MATHS NCERT, SUPPLEMENTARY EXERCISE So to make this form u have to multiply nd divide by 5, then ur answer will be 5Evaluate the following limits, if exist lim(x→0) (log(1x3))/sin3x



Doubt From Limits Maths Doubts Goiit Com


How To Evaluate Math Lim X To Infty X 1 X Math Quora
For specifying a limit argument x and point of approach a, type "x > a" For a directional limit, use either the or – sign, or plain English, such as "left," "above," "right" or "below" limit sin(x)/x as x > 0;How to use the limit of Limit of (log a (1 x))/x to solve the given limit with a logarithmic function formula, 1 example, and its solution Formula The limit of (log a (1 x))/x as x → 0 is 1/(ln a)Integrate log(1/x – 1) upper limit =1 lower limit=0 Dear Suraj log (1/(x1)) = Log 1 log (x1) = 0 log (x1) Now integrate it by byparts And then put limit



Evaluating Limits A Plus Topper


Find The Limit As X Goes To 0 Of 1 X 1 X E X Stumbling Robot
The logarithm log b (x) = y is read as log base b of x is equals to y Please note that the base of log number b must be greater than 0 and must not be equal to 1 And the number (x) which we are calculating log base of (b) must be a positive real number For example log 2 of 8 is equal to 3Get an answer for 'how to integrate log({sqrt(1x)}{sqrt(1x)} where lower limit is 0 and upper limit is 1' and find homework help for other Math questions at eNotesSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more



Solved Find The Following Limit Lim X Rightarrow 0 Ln 1 X X X 2 1 Answer Transtutors



Chan Sovannara Limit Facebook
What is the integration of log (1x/x) with limit 0 to 1?Exponential and Logarithmic Function and Series,Expansion of e^x,a^x and log(1x) xx Graph for exponential function Sourcewwwsheloves mathcomDec 17, 19 · lim(x →0) (xcosx log(1 x))/x2 is equal to (A) 1/2 (B) 1 (C) 0 (D) 3 lim(x →0) (xcosx log(1 x))/x^2 is equal to (A) 1/2 (B) 1 ← Prev Question Next



Rudin Ch 8 Pdf Document



Answered 17 A If Y Log X Use Differentiation Bartleby
Jul 31, 16 · We can take the natural log of something and then raise it as the exponent of the exponential function without changing its value as these are inverse operations but it allows us to use the rules of logs in a beneficial way lim_(xrarroo) (ln(x))^(1/x) = lim_(xrarroo) exp(ln((ln(x))^(1/x))) Using the exponent rule of logs =lim_(xrarroo) exp(1/xln(ln(x))) Notice thatX1x≤log(1x)≤x or similar inequalities I cannot use derivatives, Taylor expansion, o(x),O(x) and similar things Using the inequality that I have written above and the substitution x=1sint I have been only able to prove that the limit is greater or equal than 0 and smaller or equal than 1Lim x0 logx/x1 = log (0)/1 but log of zero is not defined using l hospitals rule to solve indeterminate form lim x> o (d (log x )/dx) / (d (x1)/dx) = lim x>0 (1\x) /1 ————— (1) = 1/0 which is undefined 3K views Ravi Ranjan Kumar Singh
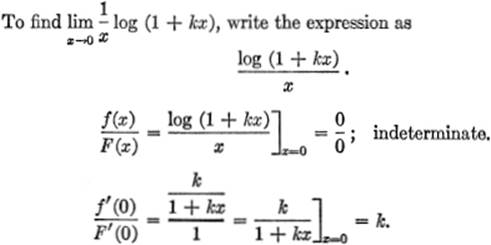


Evaluation Of Indeterminate Forms Indeterminate Forms The Calculus Primer
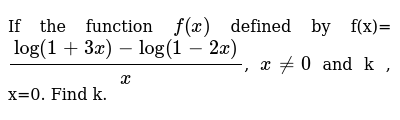


If The Function F X Defined By F X Log 1 3x Log 1 2x
Ln y = x → 0 lim x ln x = x → 0 lim 1 / x ln x = L'H x → 0 lim − 1 / x 2 1 / x = x → 0 lim − x = 0 Hence, y = e 0 = 1 y = e^0 = 1 y = e 0 = 1 Yes, it is correct No, it is incorrectJun 18, 18 · lim f(x) →0 ln(1 f (x)) f (x) = 1 to emphasize even more that fact Anyway, on with the computation let's break the fraction in the sum of two fractions ln(1 ax) log(1 bx) x = ln(1 ax) x log(1 bx) x We now multiply each fraction by 1, using the obvious equalities 1 = a a = b b the expression becomesThe natural logarithmic limit rule is a most useful formula for dealing the logarithmic functions in the limits According to this limit formula, the limit of a rational expression in the following form is equal to one lim x → 0 log e ( 1 x) x = 1 Now, let us learn how to derive the natural logarithmic limit rule in calculus mathematically in three steps


What Is The Answer To Math Lim X To 0 Bigl Frac Tan X X Bigr 1 X 2 Math Quora



Show That Limit Of Log 2x 3 2 X 2 1 As X Approaches To 2 Brainly In
1 Here, $ x $ denotes the greatest integer less than or equal to $ x $ Given that $ f(x) = x x $ The value obtained when this function is integrated with respect to $ x $ with lower limit as $ \frac{3}{2} $ and upper limit as $ \frac{9}{2} $ , isWe want to figure out the limit the limit as X approaches 1 of the expression x over X minus 1 minus 1 over the natural log of X so let's just see what happens when we just try to plug in the 1 what happens if we evaluate this expression at 1 well then we're going to get we're going to get a 1 here over 1 minus 1 so we're going to get something like a 1 over a 0 minus 1 over and what's theIn this tutorial we shall discuss the very important formula of limits, \\mathop {\lim }\limits_{x \to \infty } {\left( {1 \frac{1}{x}} \right)^x} = e\ Let us



Limits Continuity Differentiation Theory



Solved 30 Ininx In 1 X In Problems 31 34 Evaluate T Chegg Com
Specify Method \log 1 2 3\pi e x^{\square} 0 \bold{=} Go {x1}\right) en Related Symbolab blog posts Advanced Math Solutions – Limits Calculator, the basics The limit of a function is a fundamental concept in calculus concerning theHow to show the limit of (1x)^(1/x) is equal to the constant 'e'Begin by letting a variable equal to the limit, then apply the natural logarithm to both sidAs a consequence, log b (x) diverges to infinity (gets bigger than any given number) if x grows to infinity, provided that b is greater than one In that case, log b (x) is an increasing function For b < 1, log b (x) tends to minus infinity instead When x approaches zero, log b x goes to minus infinity for b > 1 (plus infinity for b < 1



Lim X Tends To 0 Loge 1 5x X Brainly In


Functions Limits And Continuity
May 01, 12 · So lim x>0 ln (1x) / x = lim x> (1/ (1x)) / 1 = 1 Alternatively, for small x ln (1x) ≈ x x^2 / 2 x^3/3 So the fraction is 1 x/2 x^2/3 , which clearly goes to 1 for x to zero Show more alwbsok Lv 7 9 years ago log (1 x) / x$\begingroup$ $\log(1x)$ is not defined (except in complex sense) when $x>1$ That explains the Mathematica result Because the "limit" is $\log(1) = i\pi$ $\endgroup$ –Limits involving ln(x) We can use the rules of logarithms given above to derive the following information about limits lim x!1 lnx = 1;


Evaluate The Limit Limx 0 Log 1 X 3 X 1 Sarthaks Econnect Largest Online Education Community



Prove The Limit As X Tends To 0 Of Log 1 X X Is 1 Brainly In
X approaches infinity The limit of the logarithm of x when x approaches infinity is infinity lim log 10 (x) = ∞ x→∞ x approaches minus infinity The opposite case, the logarithm of minus infinity (∞) is undefined for real numbers, since the logarithmic function is undefined for negative numbers lim log 10 (x) is undefined xStep 1 Enter the limit you want to find into the editor or submit the example problem The Limit Calculator supports find a limit as x approaches any number including infinity The calculator will use the best method available so try out a lot of different types of problems You can also get a better visual and understanding of the function byNov 06, 15 · In two different ways, prove the limit as x tends to 0 of (log (1x))/x is 1 by RoRi November 6, 15 The following limit equation is valid Prove this in the following two ways Using the definition of the derivative ;


Limit Log X 1 X



Evaluate Lim X Tends To 1 Log 1 X 2 Cotpx Brainly In
Get the free "Log(1x) Taylor Series" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Mathematics widgets in WolframAlphaFormula lim x → 0 ln ( 1 x) x = 1 The limit of the quotient of natural logarithm of one plus a variable by the variable as the input approaches zero is equal to one It is called the natural logarithmic limit ruleLimits to Infinity Calculator online with solution and steps Detailed step by step solutions to your Limits to Infinity problems online with our math solver and calculator Solved exercises of Limits



Limits Ex 29 10 Q23 Log 3 X Log 3 X Lim 3 X Lim X 0 2x 3 X Lim X 2x Log 1 3 X Lim X Lim 2x 3 X 2x 63 X X 03 X


Solved Compute The Following Limits Lim X Rightarrow 0 Chegg Com
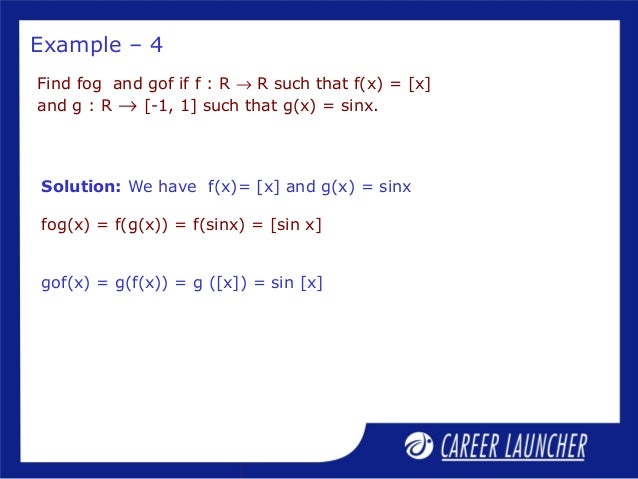
Find the answer to this question along with unlimited Maths questions and prepare better for JEE exam


Finding Derivative Of X X Using Limit Definition Physics Forums
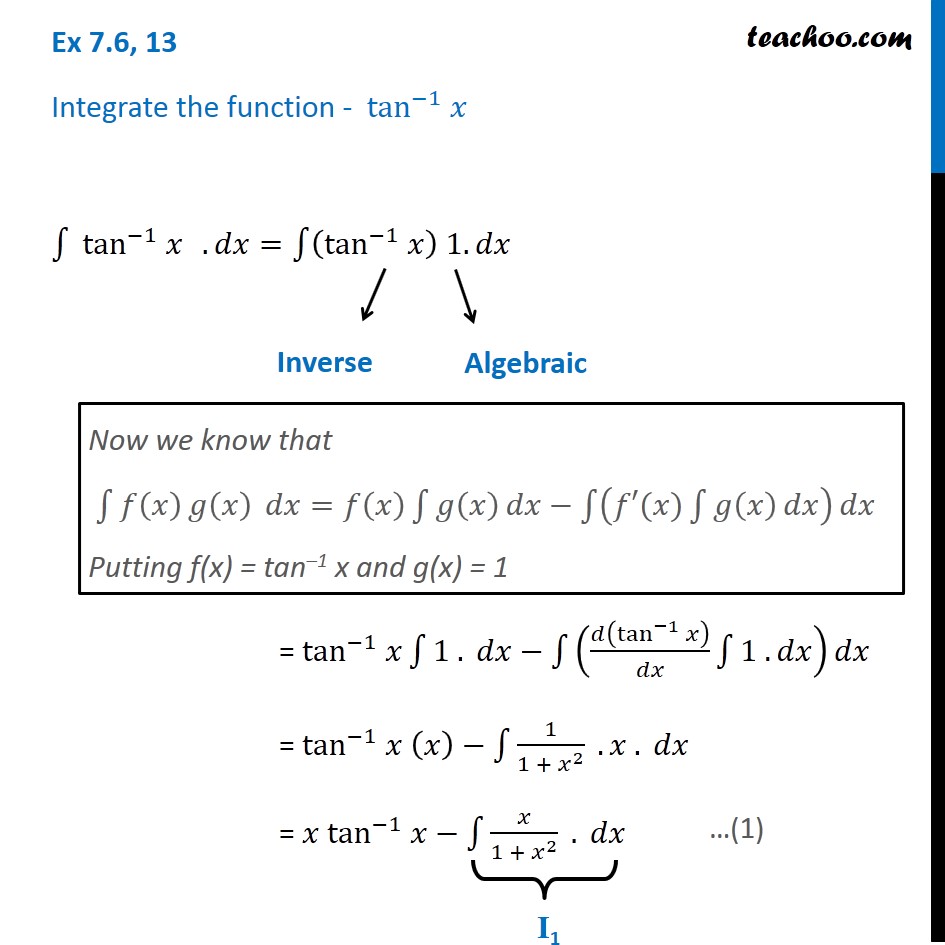


Integration Of Tan Inverse X Ex 7 6 13 Chapter 7 Class 12
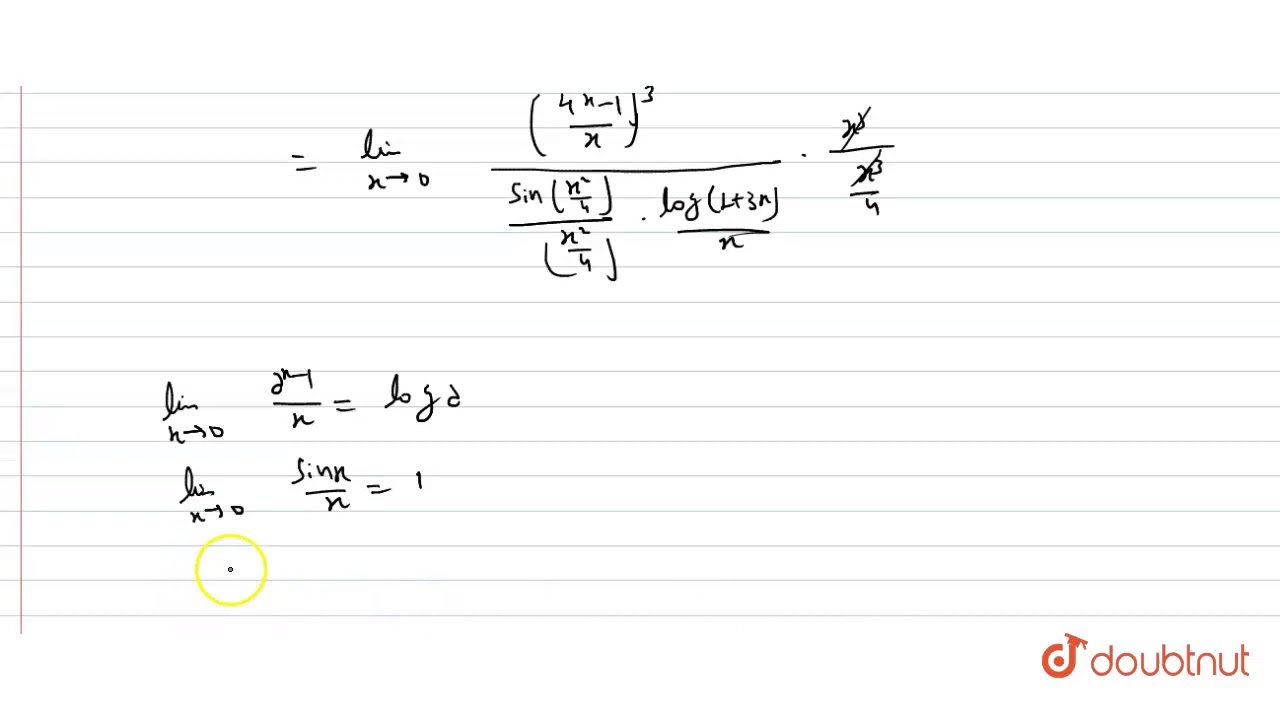


Evaluate The Following Limit Lim 4 X 1 3 Sin X 2 4 Log 1 3x Youtube
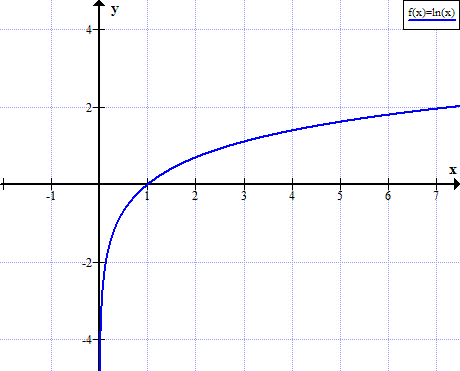


Natural Logarithm Rules Ln X Rules
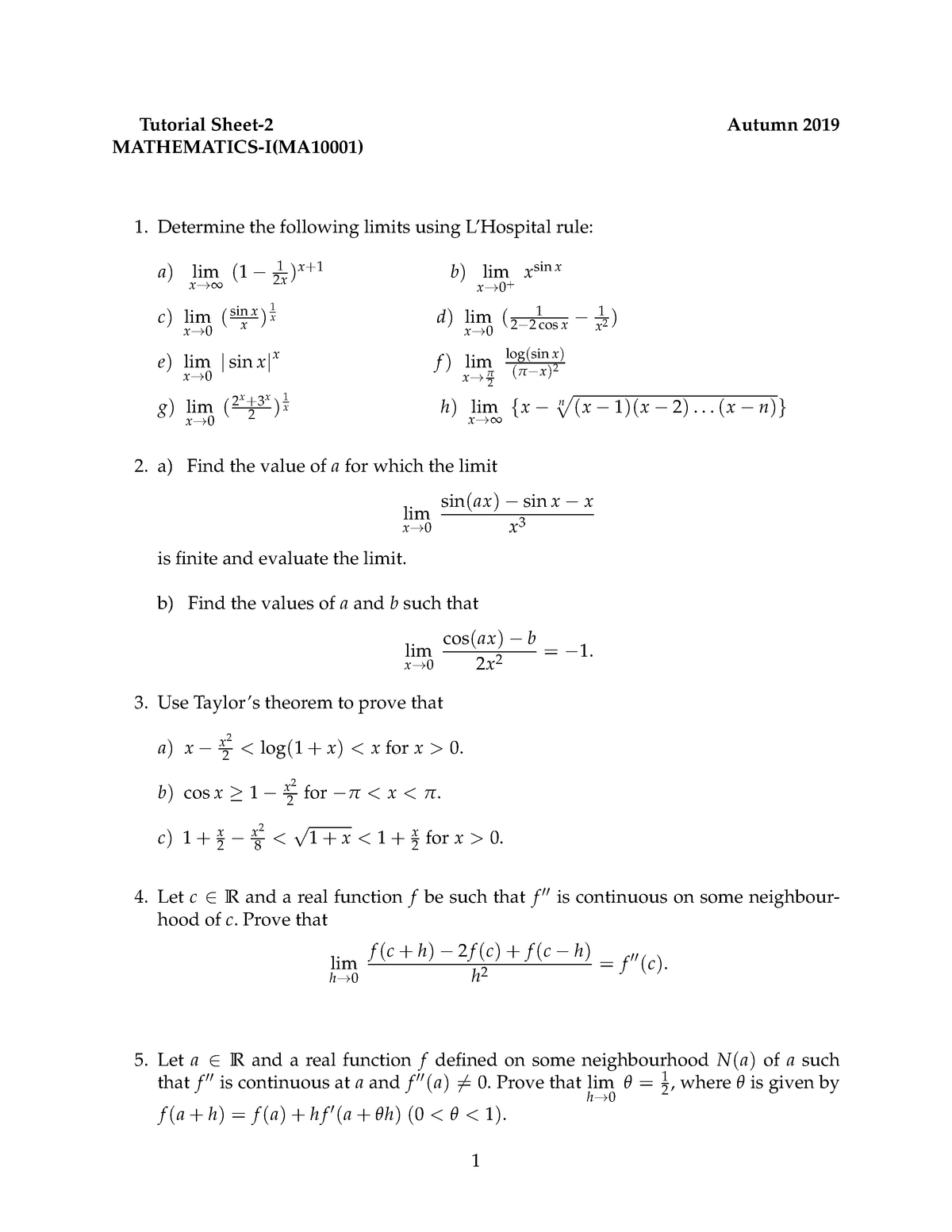


Tutorial Sheet 2 Lorem Ipsum Is Simply Dummy Text Of The Printing And Typesetting Industry Lorem Studocu


What Is The Value Of Sin X Log 1 X X 2 Limit X Tending To 0 Quora


Evaluate The Following Limits If Exist Lim X 0 Log 1 X 3 Sin 3x Sarthaks Econnect Largest Online Education Community



What Is The Limit Of Ln X 1 X When X Approaches 0 Quora



How Do You Find The Limit Of Ln X 1 X As X Approaches Infinity Socratic



Limit As X Approaches Infinity For Log Of Greatest Integer Over X Youtube



Limit X Tends To 0 Log 5 X Log 5 X X Maths Meritnation Com


Find The Limit As X Goes To 1 Of 1 Log X 1 X 1 Stumbling Robot



Natural Log Limit E In Denominator Page 5 Line 17qq Com



Lim X 0 Sin Log 1 X Log 1 Sinx Of Lim Sin Log I X X 0



Explicit Expression For 1 E As A Limit Mathematics Stack Exchange



Solved Exercice1 A Compute The Following Limits Log X2 Chegg Com


Find The Limit As X Goes To 0 Of Ax 1 Bx 1 Stumbling Robot



Asymptotic Limit Of Truncated Legendre Sieve Mathoverflow



Problem In Realizing Aep Like Limit Mathematics Stack Exchange


In Two Different Ways Prove The Limit As X Tends To 0 Of Log 1 X X Is 1 Stumbling Robot
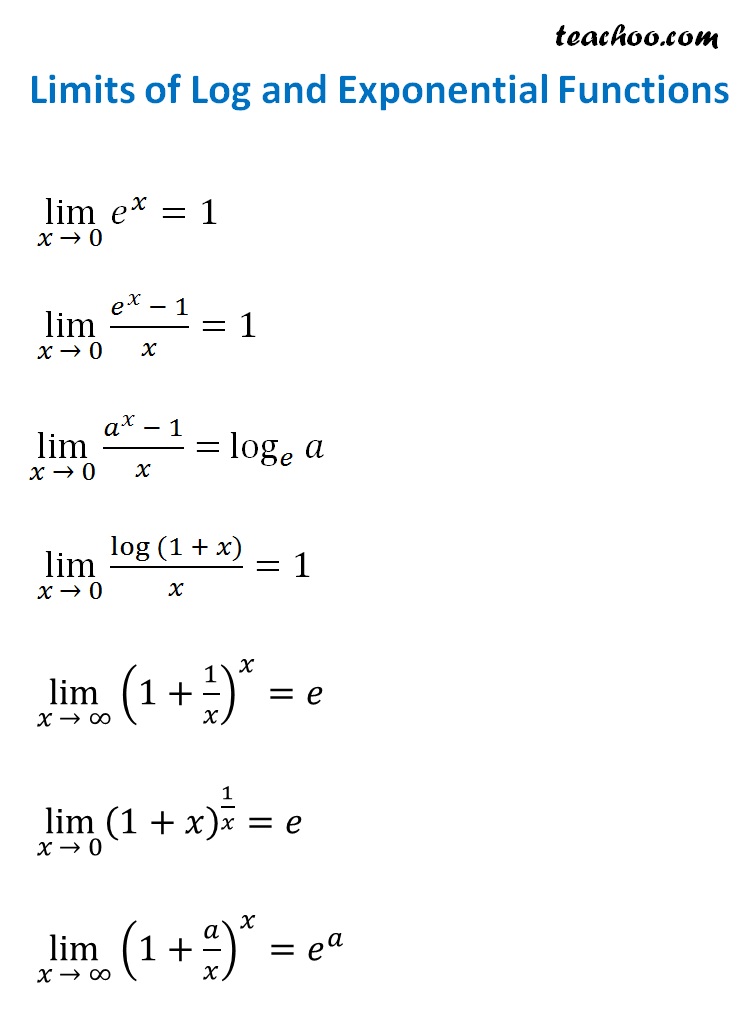


Limits Formula Sheet Chapter 13 Class 11 Maths Formulas Teachoo
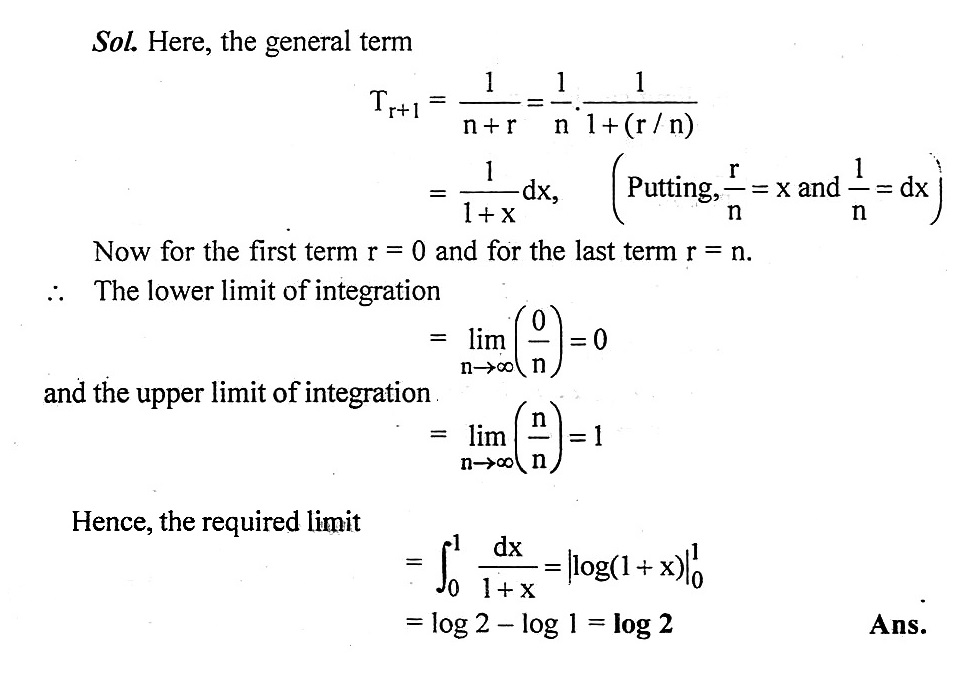


Evaluate Lim N 1 N 1 1 N 2 1 2n Mathematics 1 Question Answer Collection



Definition Of Derivatives To Prove Limit Ln 1 X X 1 Youtube


How To Prove Lim X To Infty Frac Log 1 F X F X 1 Without Using L Hopital S Rule Mathematics Stack Exchange



Lim X To 0 Frac Pi X Log X Pi X 2 X 2 1 2 Should Not Be Defined Right Mathematics Stack Exchange



Prove That Lim X 0 Log E 1 X X 1 Maths Limits And Derivatives Meritnation Com


Lim X Approaches To 0 Gx F X Mathhomeworkanswers Org


Integration Of Logx Lim 1 E To E Brainly In



E Mathematical Constant Wikipedia


Doubt From Log Expansion Maths Doubts Goiit Com



Integrate Log 1 X 1 X2 Dx Upper Limit 1 Lower Limit 0 Maths Integrals Meritnation Com



Lim X 0 Sin 3 Sqrtx Log 1 3x Tan 1 Sqrtx 2 E 5sqrtx 1



Limit X Gt 0 Log 1 X 1 X X 2 1 X Options A 1 2 B 1 2 C 0 D 1 Mathematics Topperlearning Com Ykn6m377



Integration Of Tan Inverse X Ex 7 6 13 Chapter 7 Class 12



Lim X Tends To 0 Log 1 2x Tanx Brainly In


Evaluate Lim X 0 Log 1 3x Log 1 2x Sarthaks Econnect Largest Online Education Community



Lim X Tends To 0 Log 6 X Log 6 X X Log 6 X A Log 6 X 80 Lim 1 2 Solution We Have Maths Limits And Derivatives Meritnation Com


Lesson 25 Indeterminate Forms And L Hopital S Rule



Evaluate The Limit Log Log F X Log X Mathematics Stack Exchange


Limits And Continuity Basic And Conceptual Maths With Prashant Karn


Find The Limit As X Goes To 1 Of Log X X2 X 2 Stumbling Robot


Evaluate The Following Limit Lim Sin E X 2 1 Log X 1 Youtube
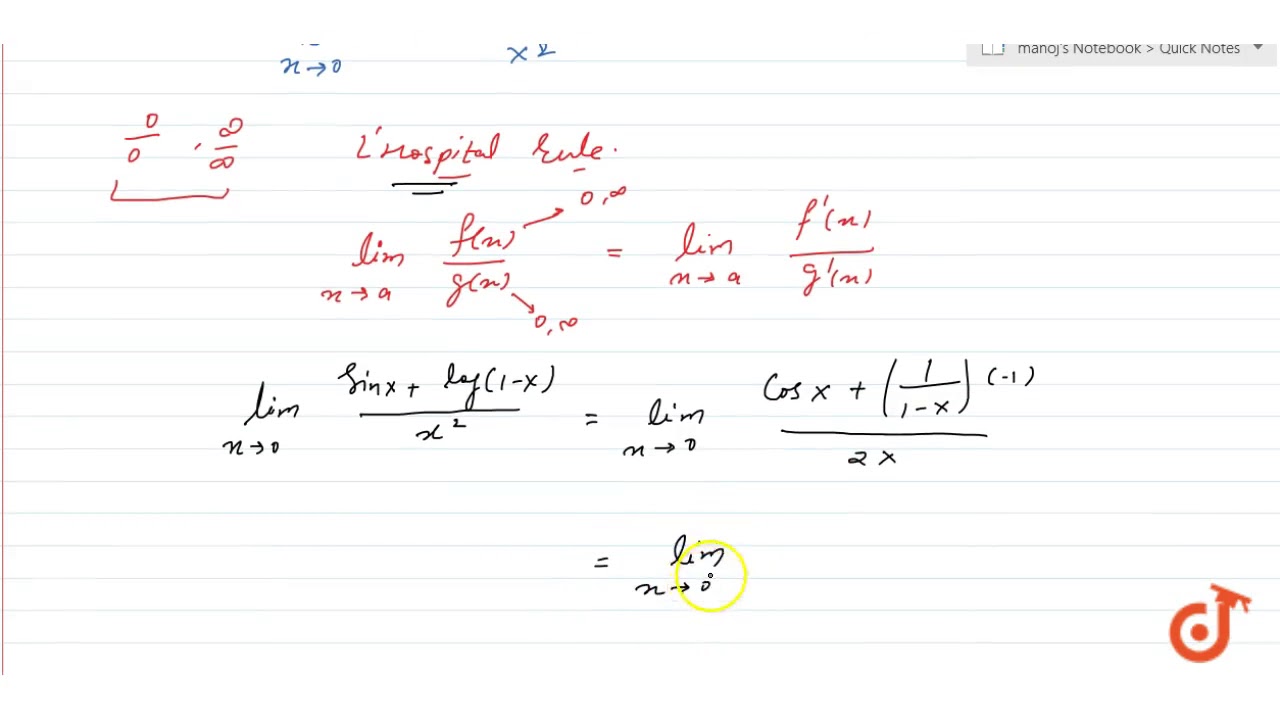


Lim X Gt0 Sinx Log 1 X X 2 Youtube


Evaluate Lim X 0 1 X Log 1 X X 2 Sarthaks Econnect Largest Online Education Community
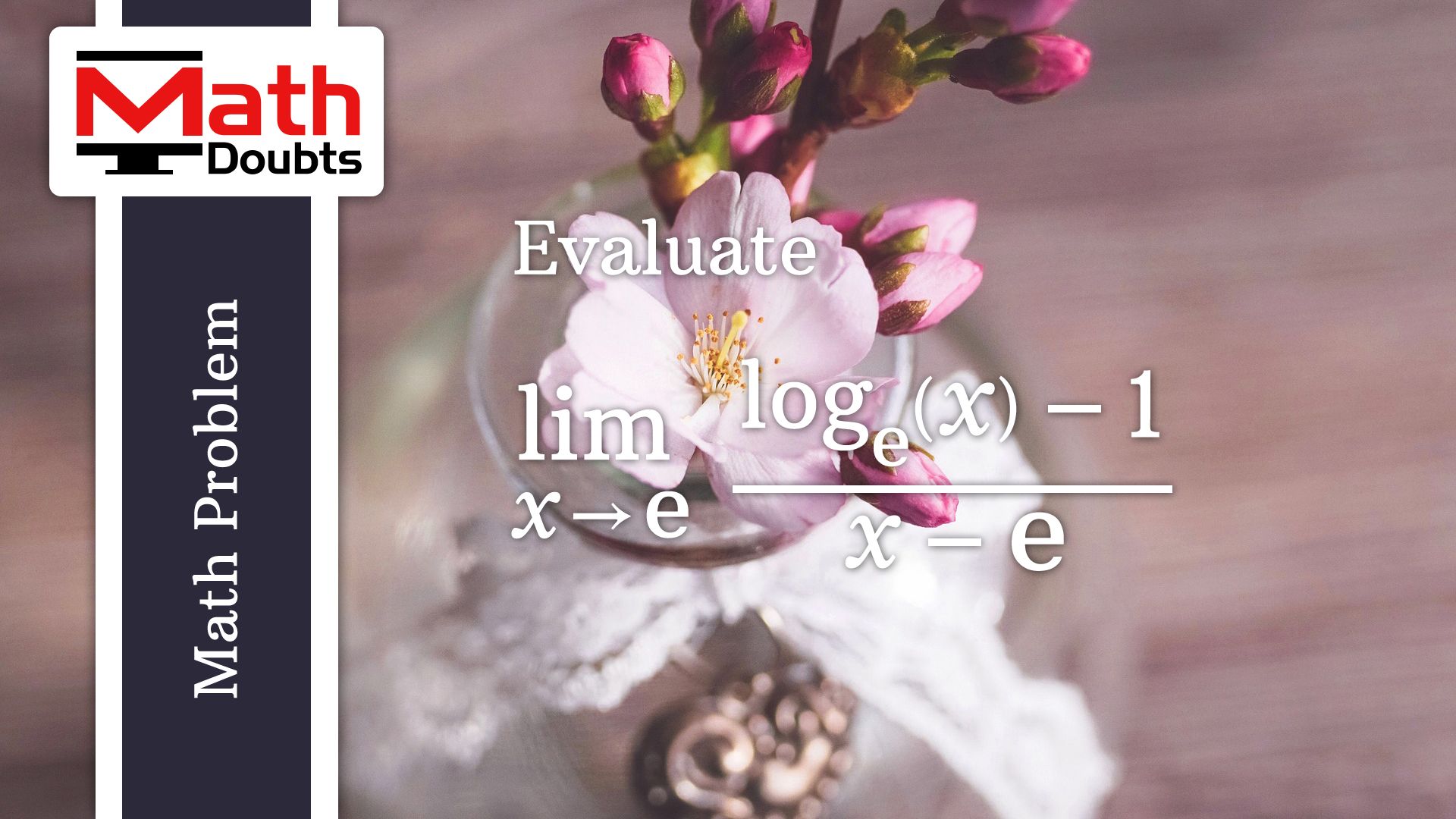


Evaluate Lim X E Lnx 1 X E Limits Calculus Evaluation Problem And Solution


What Is The Limit Of Log X 1 1 X As X Approaches To 0 Quora


Taylor Series For Log X Physics Forums


Find The Limit As X Goes To 0 Of Log 1 X E2x 1 Stumbling Robot



Finding The Limit As X Goes To A Of Log X A Mathematics Stack Exchange



How To Evaluate Lim X To Infty Left 1 Frac 2 X Right 3x Using L Hopital S Rule Mathematics Stack Exchange



Lim X 1 X 1 Log X Mathematics Topperlearning Com 9gqsqoii



Solved Or Every Ned 4 4 5 Evaluate The Following Limits Chegg Com



Prime Number Theorem Wikipedia



Let F X 3x 4tanxx For X 0 7 For X 0 Then F X Is



Evaluate Lim X E Logx 1 X E
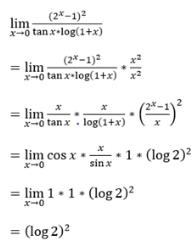


Test Infinite Limits 10 Questions Mcq Test


Evaluate Lim X 0 Xe X Log 1 X X 2 Sarthaks Econnect Largest Online Education Community



Q No Iii Plz Answer Fast Ii Prove Lim Log 1 2x 2 Aœ Ii Sin3x 3 C Iii Maths Limits And Derivatives Meritnation Com


Evaluate The Following Limits If Exist Lim X 0 Log 1 2x X Sarthaks Econnect Largest Online Education Community



How To Evaluate The Limit Math Lim N To Infty Left Frac N N N Right 1 N Math Quora



Answered 1 Find The Following Limits r Lim Bartleby


Gate Cse 15 Set 3 Question 9 Gate Overflow



Solved Find F X If F X Log X 3 1 X 3 6 Write Chegg Com
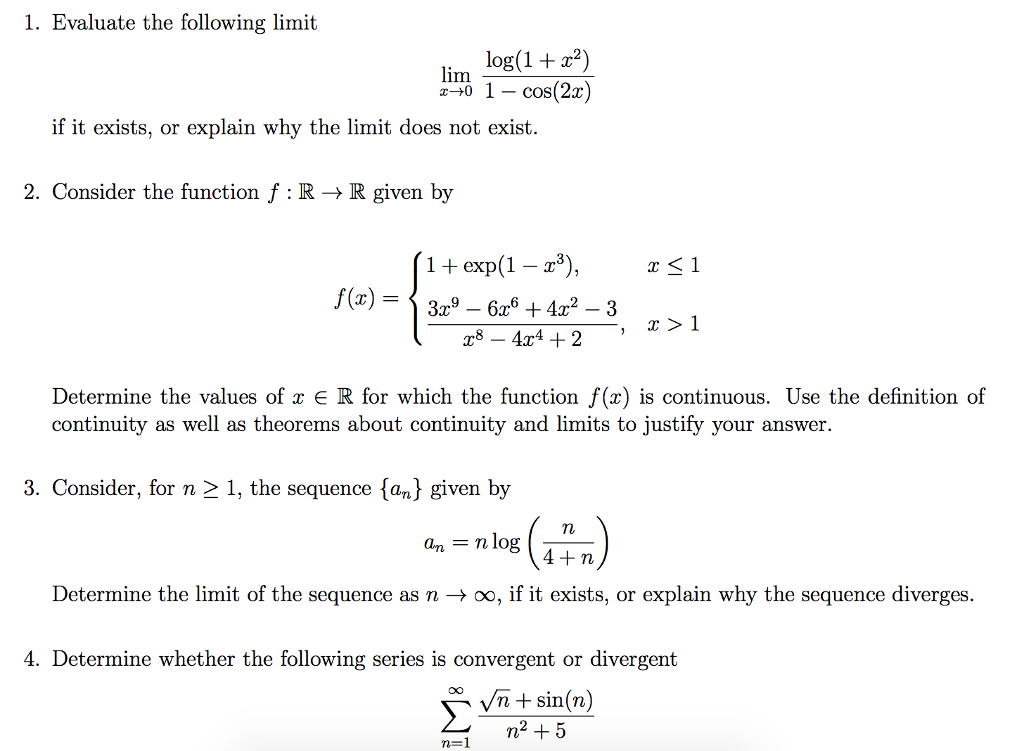


Solved Evaluate The Following Limit Lim X Rightarrow 0 Lo Chegg Com



Proof Of Lim X 0 Log 1 X X 1 Youtube



対数の極限でlog と Limの扱いについて 数学 大学 に関する質問 勉強質問サイト



Example 40 Evaluate Log Log X 1 Log X 2 Dx Examples



1 5 Marks Let F X A Use L Hopital S Rule To Evaluate The Limit As 2 Homeworklib



How To Count The Limits The Types Of Uncertainty Limits Math


Some Important Other Limits Mathematics



Lim X 1 Logx X 1


コメント
コメントを投稿